Current Monthly Trading Article

As we near the end of October, you should be aware of two important market cycles: the six-month cycle and the Presidential cycle. Entering November, they reach a bullish confluence that historically has proved very profitable. As the names imply, the six-month cycle divides the year into halves, and the Presidential cycle segregates annual market returns by where they fall in the national election cycle. Yale Hirsch has written extensively about these and other cycles in his “Stock Trader’s Almanac.”

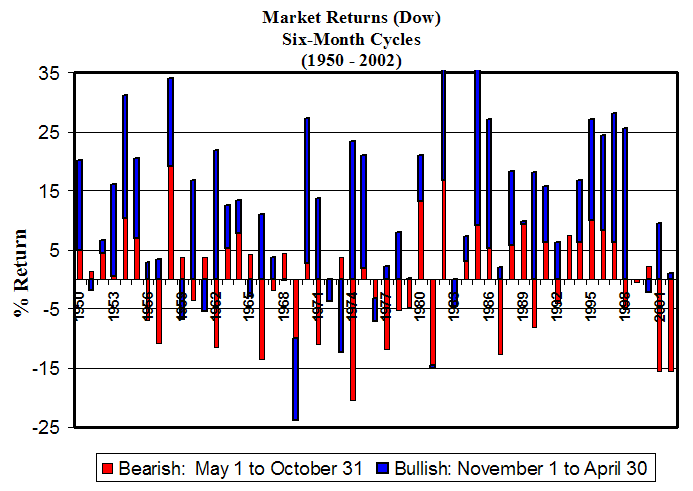
For the Dow, if you had invested $10,000 in 1950 independently in the May 1-to-Oct 31 and Nov 1-to-Apr 30 periods—taking your money out of the market when the respective six-month period was done—by Oct 24, 2003, you would have only $9,466 remaining in the first account and $471,355 in the second. Further, you would have been exposed to far less market risk being out of the market for six months each year. This contrast is understandable in terms of market liquidity. During the second period, money flows into the market: most companies end their fiscal year; mutual funds distribute; Christmas puts money into the economy; companies pay end-of-the-year bonuses; early in the year, people receive tax refunds. The bearish period, on the other hand, includes the summer doldrums, generally a period of lower volume in the market as the institutional professionals vacation.

For whatever reason, there is a huge difference in performance between the two periods. My question with these cycles is, “Are these differences significant?” That is, what is the likelihood that this distribution of returns could have occurred by chance alone? If the pattern is likely to occur by chance, then the historical differences can be expected to bear no relation to future returns. I used a resampling approach to assess the significance of these differences. Under the assumption that no difference existed between these two halves of the year, I would expect the distribution of returns to show no preference for either half-year. Of course, if that were true, then past trends would not necessarily continue into the future. In the same way, flipping a fair coin and getting heads ten times in a row, though admittedly a rare event, does not improve its odds from the 50/50 chance of getting heads on the next flip.

The 106 biannual returns were randomly distributed by computer resampling; these gains were again segregated by the half-year; then their respective six-month periods summed. Finally, the 53 year biannual sums were compared to the 426% gain actual obtained in the Nov-to-Apr period. Only two times in 100,000 such random redistributions did a summed return exceed 426%, which shows that the observed biannual distribution is indeed a rare event; the half-year cycle is for real, and there are definitely good and bad halves of the year for investment.

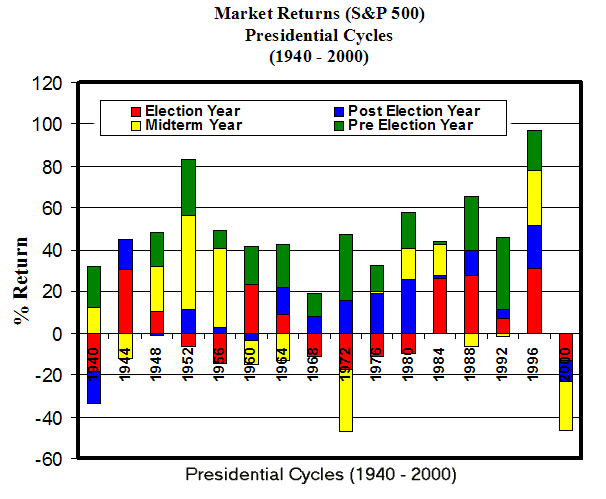
The second cycle, the Presidential cycle, segregates annual market returns by where they fall in the national election cycles. From the 1940 Roosevelt cycle through the 2000 Bush cycle, 16 annual returns of the S&P 500 have summed thusly: 118.9% for election year (next 2004), 63.1% for post-election year (next 2005), 76.6% for midterm year (next 2006), and 263.7% for pre-election year (current 2003),. Further, 12 of 16 years have produced positive returns for the election year, 8 of 16 for the post-election year, 9 of 16 for the midterm year, and 15 of 15 years for the pre-election year (we’re currently in the 16th). Clearly, the pre-election year has performed far better than the other three. And that makes sense as the incumbent party does everything it can to hold power. But again, there’s the significance question. To be useful, this distribution must be significantly different from what one might expect form random redistribution.

As before, a resampling approach can be used to address the question of significance. After randomly redistributing these 63 annual returns into four-year cycles 10,000 times, only 50 times did a total return exceed 263.7% for one of the cycle’s years. Again, this is a very rare event and means that the observed distribution is very significant. Note: 6,038 times returns exceeded 118.9%, 8,372 times returns exceeded 76.6%, and 8,814 times returns exceeded 63.1%. The third year in an election cycle (e.g., 2003) is very bullish for the market while the other three are significantly less so (and statistically judged equivalent). Come this November, we begin a very bullish confluence of these two cycles.

Send an email to rmiller@triplescreenmethod.com.